Same Q – Same P-R
The key notion here is that the quality of a transformation performed by an activity Q is determined by its facilitation expressed in the form of environmental conditions. The conditions may have a positive effect as well as a negative effect on the transformation. Typically, better facilitation leads to better results, and the same holds for the opposite. In any case, a change in conditions means a change in the system in which Q is contained without changing the reason for existence (P-R).
In practice, this usually means that if more resources are allocated for a system, the better a system can perform. For instance, a school might invest in more teachers resulting in smaller class sizes eventually leading to a higher quality of education. On the other hand, if less resources are available, this might have the negative effect that the quality of education is reduced to an extent that new student registrations are lagging behind. This is a reinforcing, downward-oriented loop. In the end, the school has to close its doors because its reason for existence – educating students – disappeared due to lack of students.
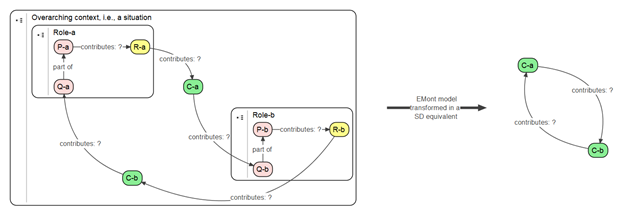
The “same Q – same P-R” pattern is akin to the hard systems approach of Systems Dynamics (SD). Think of conditions as variables and regard activities and goals as given but not required for modeling purposes, the resulting network of conditions then resembles a SD model. So, SD kind of modeling is implicitly supported within EMont. Even SD stocks and flows can be modeled in EMont. The EMont element Outcome is used as a means to explicate what is being produced or consumed by an activity. By refining the Outcome element and the produces and consumes relations by semantic web principles, they can be turned into a SD stock and flow construct. A computational model for EMont is given in EMont interpretation that shows how EMont models can be executed.
Note that by eliminating all EMont elements but the conditions, the resulting SD model resembles, but is not identical to the LoF expression [math]\displaystyle{ \overline{\underline{\overline{_{\lfloor}a|}b}\Big|} }[/math]. For one thing, the SD model and the LoF expression are based on a radical different system conception. The crucial concept that is missing in the SD model is the notion of re-entering the [math]\displaystyle{ \overline{a|}b }[/math] (a implies b) or the [math]\displaystyle{ \overline{b}a }[/math] (b implies a) distinction in their own indicational spaces to determine a next step based on past behavior (see Self-Reference in a Three-Valued System).
- Lees hiervoor:
- Lees hierna: